Let’s discuss the question: show that the equation has exactly one real root 2x-1-sinx. We summarize all relevant answers in section Q&A of website Activegaliano.org in category: Blog Marketing. See more related questions in the comments below.

How do you show that an equation has exactly one real root?
To prove that the equation has at least one real root, we will rewrite the equation as a function, then find a value of x that makes the function negative, and one that makes the function positive. . The function f is continuous because it is the sum or difference of a continuous inverse trig function and a polynomial.
How do you prove that a function has only one zero?
exactly one zero at least one zero in the given interval. Rolle’s Theorem shows that there cannot be two points x = a and x=b for which f(a) = f(b) in this interval, the function f(x) has exactly one zero in the interval [-1, 0].
Rolle’s Theorem to Prove Exactly one root for Cubic Function AP Calculus
Images related to the topicRolle’s Theorem to Prove Exactly one root for Cubic Function AP Calculus

What does Rolles theorem say?
Rolle’s theorem, in analysis, special case of the mean-value theorem of differential calculus. Rolle’s theorem states that if a function f is continuous on the closed interval [a, b] and differentiable on the open interval (a, b) such that f(a) = f(b), then f′(x) = 0 for some x with a ≤ x ≤ b.
Which equation has exactly one real solution?
A quadratic equation has exactly one real number solution.
How do you know if an equation has exactly one solution?
Equations with one solution
Some equations have exactly one solution. In these equations, there is only one value for the variable that makes the equation true. You can tell that an equation has one solution if you solve the equation and get a variable equal to a number.
What theorem can be used to determine whether a function has any zeros in a given interval?
Summary: Intermediate value theorem can be used to determine whether a function f(x) has any zeros in a given interval.
Prove the equation has at least one real root (KristaKingMath)
Images related to the topicProve the equation has at least one real root (KristaKingMath)
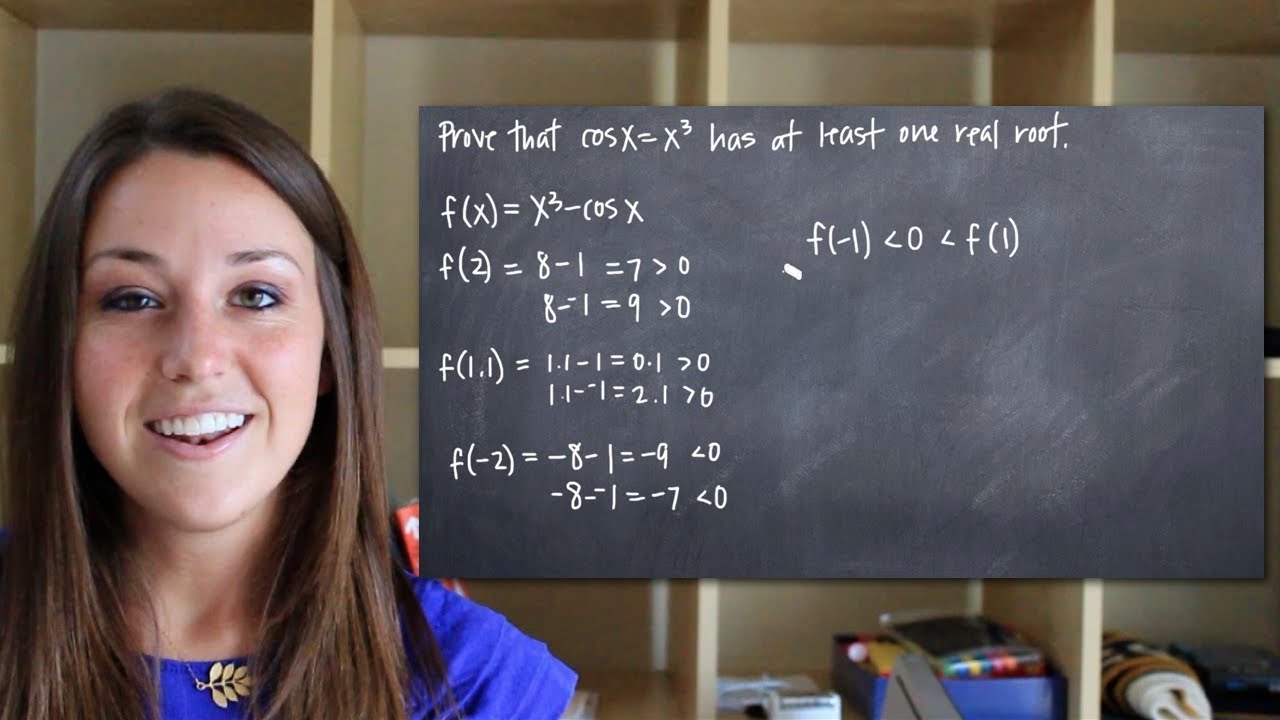
How do you use the intermediate value theorem?
- Define a function y=f(x).
- Define a number (y-value) m.
- Establish that f is continuous.
- Choose an interval a,b].
- Establish that m is between f(a) and f(b).
- Now invoke the conclusion of the Intermediate Value Theorem.
Why is Rolles theorem a special case of mean value theorem?
(The Mean Value Theorem claims the existence of a point at which the tangent is parallel to the secant joining (a, f(a)) and (b, f(b)). Rolle’s theorem is clearly a particular case of the MVT in which f satisfies an additional condition, f(a) = f(b).)
How do you make Rolles?
Rolle’s Theorem states that if f is a continuous function on the closed interval [a,b], differentiable in the open interval (a,b), and f(a)=f(b) then there exists at least one number c in (a,b) such that the f'(c) = 0.
What is the conclusion of Rolles theorem?
The conclusion of Rolle’s Theorem says there is a c in (0,5) with f'(c)=0 .
What is an example of a one solution equation?
Linear Equations With one Solution
Example 1: Consider the equation 7x – 35 = 0. On solving we have 7x = 35 or x = 5. The above linear equation is only true if x = 5 and hence the given linear equation has only one solution i.e. x = 5.
Show that the equation x² +3x+1=0 has exactly one real root solution
Images related to the topicShow that the equation x² +3x+1=0 has exactly one real root solution
Which graph shows exactly one solution?
If the graphs of the equations intersect, then there is one solution that is true for both equations. If the graphs of the equations do not intersect (for example, if they are parallel), then there are no solutions that are true for both equations.
What is the intermediate value theorem formula?
The Intermediate Value Theorem (IVT) is a precise mathematical statement (theorem) concerning the properties of continuous functions. The IVT states that if a function is continuous on [a, b], and if L is any number between f(a) and f(b), then there must be a value, x = c, where a < c < b, such that f(c) = L.
Related searches
- show that the equation has exactly one real root 3x cos x = 0
- show that the equation has exactly one real root 2x-1-sinx
- show that the equation sin 2x 3x e x 0 has exactly one real root
- show that the equation 3x 2 cos x 5 0 has exactly one real root
- show that the equation sin(2x)+3x+e^x=0 has exactly one real root.
- prove that the equation has at least one real root cosx x3
- show that the equation has exactly one real solution 2xcos x0
- show that the equation y x3 3×2 16 y x 3 3 x 2 16 has exactly one real root what is it
- show that the equation has exactly one real root 3x cos x 0
- show that the equation has exactly one real solution 2x+cos x=0
- show that the equation has exactly one real root 6x cos x 0
- show that the equation sin2x3xex0 has exactly one real root
- show that the equation 3x + 2 cos x + 5 = 0 has exactly one real root.
Information related to the topic show that the equation has exactly one real root 2x-1-sinx
Here are the search results of the thread show that the equation has exactly one real root 2x-1-sinx from Bing. You can read more if you want.
You have just come across an article on the topic show that the equation has exactly one real root 2x-1-sinx. If you found this article useful, please share it. Thank you very much.